Multiplication up to 4-digits by 2-digits - Maths - Learning with ��̳ Bitesize

Long multiplication

When good mathematicians are stuck on a problem, they see if they can simplify it.

In long multiplication we break the sum down into parts that we can easily answer and then add the parts together to get our answer.

Example 1

16 x 27 =

We don’t know our 16 or 27 times tables off the top of our head, so what can we do?

16 = 10 + 6, so to multiply by 16 we could multiply by 10 and multiply by 6 and add the answers together.

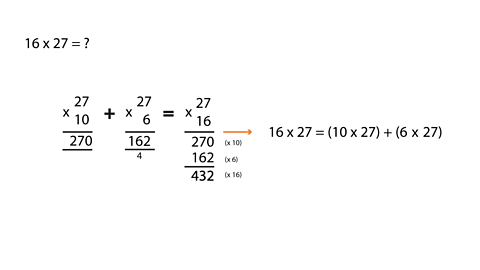
16 x 27 shown as (27 x 10) + (27 x 6) = 270 + 156 = 432

If we do the calculation the other way around we get the same answer.

27 = 20 + 7, so to multiply by 27 we could multiply by 20 (x10 and then x2) and multiply by 7.

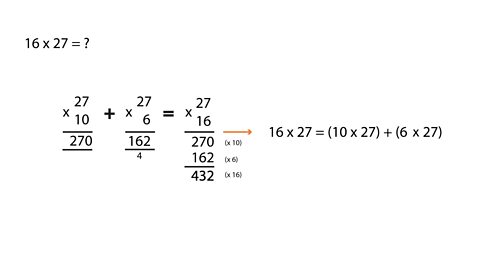
16 x 27 = 432

Example 2

3126 x 47 =

Skip image gallery

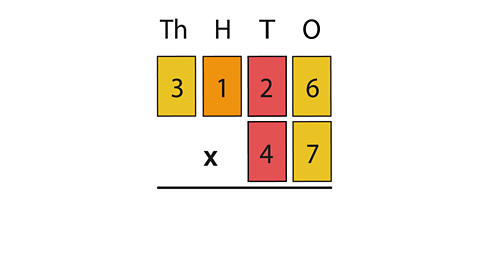
Image caption,
First we will multiply the top number by the tens (40) and then by the ones (7).
Image caption,
To multiply a number by 40, we will multiply by 10 and then by 4. Placing the zero in the ones column is the multiplying by 10 bit!
Image caption,
Now we multiply 4 x 6 = 24, so we put 4 in the tens column.
Image caption,
The 2 from 24 has carried over to the hundreds column. 4 x 2 = 8 add the 2 we carried over = 10. The 0 goes in the hundreds column and 1 is carried over to the thousands column.
Image caption,
4 x 1 = 4 then add the 1 carried over = 5, which we put in the thousands column.
Image caption,
4 x 3 = 12. 3126 x 40 = 125040
Image caption,
Now we multiply by 7. 7 x 6 = 42. Place 2 in the ones column and carry 4 over. 7 x 2 = 14 plus the 4 carried over = 18. Place 8 in the tens column and carry 1 over.
Image caption,
7 x 1 = 7 plus the 1 carried over = 8, so we put this in the hundreds column.
Image caption,
7 x 3 = 21. 3126 x 7 = 21882.
Image caption,
Now add the two answers together to get our final answer. 125040 + 21882 = 146922

Example 3

263 x 18=

Let's break this calculation into two parts again and then add together our two answers.

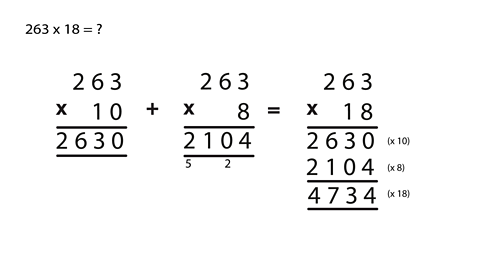
263 x 18 = 4734

Activities

Activity 1

Minimal multiplications challenge

Each player should draw 6 squares as shown in the picture below.

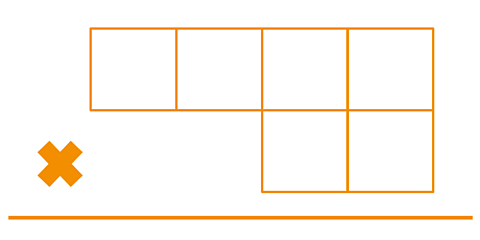
A blank multiplication grid with 4 spaces at the top and 2 spaces at the bottom

Players take it in turns to roll a 0-6 die and place each number in one of their squares.

The aim of the game is to be the player with the smallest answer to the multiplication that they have made, so think carefully about where you place the numbers! Good luck!

Extension:

If you enjoyed the game, why not try the harder version?

Play the game again, but this time when you roll the dice, you get to place the number in the other player’s grid. The aim is still to be the player with the smallest answer at the end.

How will this affect where you place the numbers compared to the first round?

Quiz

Using the methods from this lesson, try and answer the questions in this quiz.

You might need paper and pencil to help with your working out.

������̳