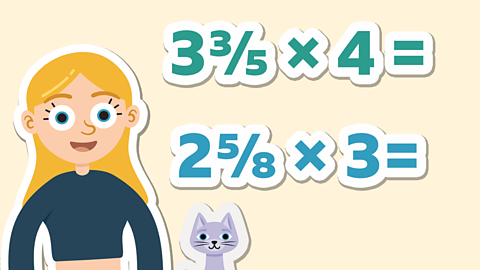
How to add mixed numbers
When adding mixed numbers, you can use a similar method to adding two fractions, but this time you have to add whole numbers as well.
Remember, a mixed number is a combination of an integer (a whole number) and a fraction, like \( 3 \frac{1}{3} \).
Example 1
What is \( 1 \frac{1}{3} + 2 \frac{2}{9}\)?
Let's break this calculation down into steps. The method below allows you to partition the mixed numbers into fractions and whole numbers, so that you can add them separately.

Step 1: Partition the mixed numbers, so you are left with the whole numbers together and the fractions together.
\( 1 + 2\)
and
\( \frac{1}{3} + \frac{2}{9} \)
You can quickly add the whole numbers together to make 3.
\( 1 + 2 = 3\)
Step 2: Focus on the fractions now.
\(\frac{1}{3} + \frac{2}{9} = ?\)
The fractions have different denominators, so you need to change one into its equivalent fraction before you add them.
You can’t simplify \( \frac{2}{9} \) any further, so you will have to change \( \frac{1}{3} \).
\( \frac{1}{3} = \frac{3}{9} \)
By multiplying the numerator and denominator by 3, you can find an equivalent fraction.
Step 3: Now that the denominators are the same, you can add the numerators together.
\( \frac{3}{9} + \frac{2}{9} = \frac{5}{9}\)
Step 4: Finally, add the answers from the whole numbers and fractions together.
\( 3 + \frac{5}{9} = 3 \frac{5}{9}\)
Example 2
Jeannie and Oliver ordered lots of pizzas. Jeannie ate \( 2 \frac{1}{4} \) pizzas and Oliver ate \( 1 \frac{1}{8} \). How much pizza did they eat altogether?

Let's write that as a calculation.
\( 2 \frac{1}{4} + 1 \frac{1}{8}\)
Step 1: Partition the fractions and whole numbers to add them separately.
\( 2 + 1 = 3\)
and
\( \frac{1}{4} + \frac{1}{8} \)
Step 2: Change one of the fractions so they both have the same denominator.
\( \frac{1}{4} = \frac{2}{8} \)
Step 3: Add the numerators together.
\( \frac{2}{8} + \frac{1}{8} = \frac{3}{8} \)
Step 4: Add the whole number answer and fraction answer together.
\( 3 + \frac{3}{8} = 3 \frac{3}{8} \)
So Jeannie and Oliver ate \( 3 \frac{3}{8} \) pizzas.
Checklist

Here’s a checklist to remind you of the steps while you work:
- Partition and add whole numbers.
- Check and change denominators.
- Add the fractions.
- Whole numbers answer + fractions answer = final answer!

Activities
Activity 1
Have a go at solving these three problems involving addition of mixed fractions.
You may need a pen and paper.
Add these mixed fractions together. Can you give the answer in its simplified form?
- 3 \(\frac {5}{8} \) + 9 \(\frac {1}{2} \)
- 8 \(\frac {4}{9} \) + 2 \(\frac {2}{6} \)
- 5 \(\frac {1}{6} \) + 6 \(\frac {1}{2} \)
On Saturday, there was 9 \(\frac {1}{6} \) hours of sunshine.On Sunday, there was 6 \(\frac {1}{5} \) hours of sunshine.How many hours of sunshine were there over the whole weekend?
Charlie has completed the calculation:
2 \(\frac {2}{6} \) + 4\(\frac{1}{4} \) = \(\frac {22}{6} \) + \(\frac{17}{4} \) = \(\frac{44}{12} \)+ \(\frac{51}{12} \) = \(\frac{95}{12} \) = 7 \(\frac{11}{2} \)
Frankie thinks he has spotted a mistake.
Can you:
- Explain the mistake Frankie has spotted.
- Find the correct answer, showing your working out.
Once you've had a go, check your here.
Quiz
Apply what you have learnt in this guide by having a go at this tap and find activity.
Play our fun maths game Guardians: Defenders of Mathematica. gamePlay our fun maths game Guardians: Defenders of Mathematica
Use your times tables and more maths skills to defeat monsters and reclaim the Kingdom of Mathematica

More on Fractions
Find out more by working through a topic
- count4 of 26

- count5 of 26

- count6 of 26
- count7 of 26